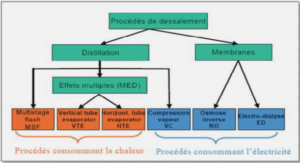
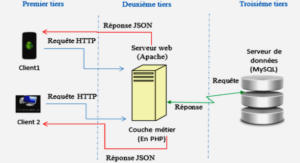
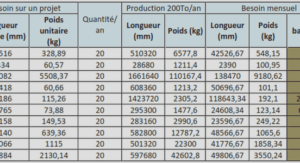
Quantication des ondes de surface [Phys. Rev. B 82, 035411 (2010)]

Dans ce chapitre, à partir d’une expression du champ des ondes de surface sous forme de somme de modes obtenue dans le chapitre 1, on détermine l’énergie associée à chacun de ces modes, puis on les quantie canoniquement, chacun d’entre-eux étant analogue à un oscillateur harmonique – comme les modes du champ électromagnétique du vide ( 2000). On obtient alors une expression des opérateurs associés au champ des ondes de surface, qui permet, dans le chapitre 5, de modéliser l’émission et l’absorption de phonons- polaritons de surface par transitions inter- et intrasousbandes des électrons d’un puits quantique. Nous serons amenés au cours de ce chapitre à dénir un facteur de Purcell associé à l’émission d’ondes de surface. Nous donnerons une expression de ce facteur de Purcell à partir du formalisme quantique que l’on développera, ainsi qu’à partir de l’expression du champ des ondes de surface dans le cas d’une fréquence complexe, obtenue au chapitre 1. Le traitement quantique négligeant les pertes des ondes de surface, une comparaison des deux facteurs de Purcell permettra d’estimer dans quelle mesure ce traitement quantique s’applique en présence de pertes.Quantum theory of light is a useful tool to describe microscopic interactions between light and matter. e electromagnetic state is represented by photon number states and the electromagnetic eld becomes an operator(L 2000). Such a description of light provides a quantitative description of absorption, spontaneous and stimulated emission of photons by a two-level system. In particular, it allows to derive a quantitative treatment of light amplication. It also predicts pure quantum effects, such as photon coalescence or antibunching. Quantum theory of light can be extended to non-dispersive and non-lossy media. Each photon in the material corresponds to the excitation of a mode characterized by a wave vector k and circular frequency !, such as k = n!/c, where n is the refractive index of the medium and c the light velocity in a vacuum. It is the purpose of this paper to introduce a quantication scheme for surface waves propagating along an interface.

It is well known that electromagnetic surface waves called surface plasmons exist at interfaces between metals and dielectrics(R 1988). eir quantum nature has been demonstrated by energy loss spectro- scopy experiments on thin metallic lms reported by Powell and Swan(t al. 2009) Surface plasmons are associated with collective oscillation of free electrons in the metal at the surface. Simi- lar electromagnetic elds exist also on polar materials and are called surface phonon-polariton. Both surface plasmon-polaritons and surface phonon-polaritons propagate along the interface and decrease in the direction perpendicular to the surface. Such a resonance is therefore called surface wave in a more general way. Most studies deal with a plane interface between air or vacuum and a non-lossy material. In this case, it is well known(R988) that a surface wave can exist if the dielectric constant ϵ(!) has a real part lower than 1. Losses are often a serious limitation for many practical applications envisionned for surface plasmons. problem could be circumvented by introducing gain in the system. Studies have been made in such a way with metallic nanoparticles embedded in a gain medium both numerically with dye molecules model stimulated emission and therefore to specify gain conditions and laser operation. It could also allow to analyse pure quantum effects for surface plasmons such as single plasmon interferences, quantum corre- lations In their work, the metal is characte- rized by a non-lossy Drude model so that real optical properties cannot be included. Using Green’s approach, Gruner and Welsch introduce a quantization scheme for electromagnetic elds in dispersive and absorptive materials (996). It should hence be possible to quantize the eld associated with sur- face waves using their model. Note that due to losses, they cannot obtain operators for modes but only local operators : one recovers the usual creation/annihilation operators in the limit of zero losses. A related work, reported in the early nineties by Babiker et al., dealt with the quantization of interface optical phonons in quantum well, which could appear also as a conned surface phonon in a heterostructure et al. 1993.