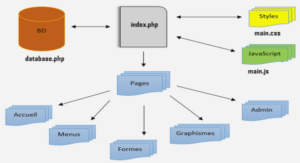
Spectral controllability for 2D and 3D linear Schrödinger equations

Definitions, notations and statement of the results 

Definition of the control problem

Let Ω be a domain of R n (i.e. a bounded non empty open subset of R n ), n ∈ {1, 2, 3}, with a C 1 boundary. We use −∆D Ω to denote the Laplacian operator on Ω with Dirichlet boundary conditions, i.e. D(∆D Ω ) = H 2 ∩ H 1 0 (Ω, C), −∆ D Ω φ = −∆φ. The space L 2 (Ω, C) has a complete orthonormal system (φk)k∈N∗ of eigenfunctions for −∆D Ω , φk ∈ H 2 ∩ H 1 0 (Ω, C), −∆ D Ω φk = λkφk, where (λk)k∈N∗ is a non-decreasing sequence of positive real numbers. With this notation, the eigenvalues λk are counted as many times as their multiplicity. For t ∈ R and q ∈ Ω, we define the function ψ1 by ψ1(t, q) := φ1(q)e −iλ1t . We recall that −i∆D Ω generates a C 0 -group of isometries of L 2 (Ω, C) defined by e −i∆tϕ := X k∈N∗ hϕ, φkie −iλktφk, ∀ϕ ∈ L 2 (Ω, C). In this paper, we study controllability properties of the linear systems (5.3) and (5.4). In order to consider them as control systems, we first need a concept of trajectories associated to these systems. For that purpose, recall that the unit sphere S of L 2 (Ω, C) is defined as follows, S := {ϕ ∈ L 2 (Ω, C); kϕkL2(Ω) = 1}, and, for ϕ ∈ S, the tangent space to the sphere S at the point ϕ is given by TSϕ :=  ξ ∈ L 2 (Ω, C); ℜ Z Ω ξ(q)ϕ(q)dq = 0 . 5.2. Definitions, notations and statement of the results 119 Définition 5.1. (Weak solutions) Let T > 0, µ ∈ C 0 (Ω, R 2 ), Ψ0 ∈ TSφ1 and v ∈ L 1 ((0, T), R n ). A weak solution to the Cauchy problem    i ∂Ψ ∂t (t, q) = −∆Ψ(t, q) − hv(t), µ(q)iψ1(t, q), (t, q) ∈ R+ × Ω, Ψ(t, q) = 0, (t, q) ∈ R+ × ∂Ω, Ψ(0) = Ψ0, (5.6) is a function Ψ ∈ C 0 ([0, T], L2 (Ω, C)) such that for every t ∈ [0, T], Ψ(t) = e i∆tΨ0 + i Z t 0 e i∆(t−s) [hv(s), µiψ1(s)]ds in L 2 (Ω, C). (5.7) Then (Ψ, v) is a trajectory of the control system (5.3) on [0, T]. Let s0, d0 ∈ R n . A weak solution to the Cauchy problem    i ∂Ψ ∂t (t, q) = −∆Ψ(t, q) − hv(t), µ(q)iψ1(t, q), (t, q) ∈ R+ × Ω, Ψ(t, q) = 0, (t, q) ∈ R+ × ∂Ω, Ψ(0) = Ψ0, s˙(t) = v(t), s(0) = s0, ˙d(t) = s(t), d(0) = d0, (5.8) is a function (Ψ, s, d) with s ∈ W1,1 ((0, T), R n ), d ∈ W2,1 ((0, T), R n ) solutions of s˙(t) = v(t) in L 1 ((0, T), R n ), s(0) = s0, ˙d(t) = s(t) in L 1 ((0, T), R n ), d(0) = d0, and Ψ ∈ C 0 ([0, T], L2 (Ω, C)) such that for every t ∈ [0, T], (5.7) holds. Then ((Ψ, s, d), v) is a trajectory of the control system (5.4) on [0, T]. The following proposition recalls a classical existence and uniqueness result for the solutions of (5.6), from which one can deduce the similar result for (5.8). Theorem 5.1. For every T > 0, Ψ0 ∈ TSφ1, v ∈ L 1 ((0, T), R n ), there exists a unique weak solution to the Cauchy problem (5.6) and Ψ(t) ∈ TSψ1(t) for every t ≥ 0. Then, the system (5.3) is a control system where – the state is the function Ψ, with Ψ(t) ∈ TSψ1(t) for every t ∈ R+, – the control is v : t ∈ R+ 7→ v(t) ∈ R n , L 1 loc(R+, R n ) is the set of admissible controls and the system (5.4) is a control system where – the state is the triple (Ψ, s, d), with Ψ(t) ∈ TSψ1(t) for every t ∈ R+, – the control is v : t ∈ R+ 7→ v(t) ∈ R n and L 1 loc(R+, R n ) is the set of admissible controls. More precisely, in this paper, we investigate the following controllability property for (5.3). Définition 5.2 (Spectral controllability for (5.3)). The system (5.3) is spectral controllable in time T if, for every Ψ0 ∈ D ∩ TSψ1(0), Ψf ∈ D ∩ TSψ1(T), there exists v ∈ L 2 ((0, T), R n ) such that the solution of (5.6) satisfies Ψ(T) = Ψf , where D := Span{φk; k ∈ N ∗ }. For the system (5.4), this definition needs to be adapted because of the presence of s and d in the state variable and because the directions ℑhΨ(t), ψ1(t)i and s(t) are linked. Indeed, any solution of (5.8) satisfies ℑhΨ(t), ψ1(t)i = ℑhΨ0, ψ1(0)i + Xn j=1 hµ (j)φ1, φ1i[s (j) (t) − s (j) (0)], ∀t, (5.9)  where, for x ∈ R n , x (j) denotes its components, x = (x (1), …, x(n) ) and h., .i denotes the L 2 (Ω, C)-scalar product. Therefore, we study the following controllability property for (5.4). Définition 5.3 (Spectral controllability for (5.4)). The system (5.4) is spectral controllable in time T if for every Ψ0 ∈ D∩TSψ1(0), Ψf ∈ D∩TSψ1(T) with ℑhΨf , ψ1(T)i = ℑhΨ0, ψ1(0)i, for every d0 ∈ R n , there exists v ∈ L 2 ((0, T), R n ) such that the solution of (5.8) with s0 = 0 satisfies (Ψ, s, d)(T) = (Ψf , 0, 0). The notations Ω, n ∈ {1, 2, 3}, φk, ψ1, h., .i, S, TS, D, x = (x (1), …, x(n) ) ∈ R n introduced in this section are used all along this article. We also denote (ej )1≤j≤n the canonical basis of R n and ωk := λk − λ1, for every k ∈ N ∗ . We use the same notation for the R n -scalar product and the L 2 (Ω)-scalar product but if a confusion is possible we precise the space in subscript h., .iL2(Ω) or h., .iRn . When some confusion is possible, we also precise the domain on the eigenvalues and eigenfunctions of the Laplacian : λ Ω k , φ Ω k . 5.2.2 Previous 1D results, difficulties of the 2D and 3D generalizations In this section, we recall classical results about the controllability of the systems (5.3) and (5.4) in 1D, that are the starting point of the strategies developed in [9] and [10] for the nonlinear systems (5.1) and (5.2). We also give their proof in order to explain the difficulties arising in their generalization to the 2D and 3D cases. We take Ω = (0, 1), so φk(q) = √ 2 sin(kπq), λk = (kπ) 2 and we use the following notations Hs (0)((0, 1), C) := D(A s/2 ) where D(A) := H2 ∩ H1 0 ((0, 1), C), Aϕ := −ϕ ′′ . 5.2.2.1 1D controllability of (5.3) For the control system (5.3), we have the following result. Proposition 5.2. Let Ω = (0, 1) and µ ∈ W3,∞((0, 1), R). (1) We assume that ∃c1, c2 > 0, c1 k 3 ≤ |hµφ1, φki| ≤ c2 k 3 , ∀k ∈ N ∗ . (5.10) Then, for every T > 0, the system (5.3) is controllable in H3 (0)((0, 1), C) with control functions in L 2 ((0, T), R) : for every T > 0, Ψ0, Ψf ∈ H3 (0)((0, 1), C) with Ψ0 ∈ TSψ1(0) and Ψf ∈ TSψ1(T), there exists v ∈ L 2 ((0, T), R) such that the solution of (5.6) satisfies Ψ(T) = Ψf . (2) We assume that there exists m ∈ N ∗ such that hµφ1, φmi = 0 and ∃c1, c2 > 0, c1 k 3 ≤ |hµφ1, φki| ≤ c2 k 3 , ∀k ∈ N ∗ such that hµφ1, φki 6= 0. (5.11) Then, the system (5.3) is not controllable : for every T > 0, Ψ0 ∈ L 2 ((0, 1), C) and v ∈ L 1 ((0, T), R) the solution of (5.6) satisfies hΨ(T), φki = hΨ0, φkie −iλkT , ∀k ∈ N ∗ such that hµφ1, φki = 0. But one can characterize the reachable set : for every T > 0, Ψ0, Ψf ∈ H3 (0)((0, 1), C) with Ψ0 ∈ TSψ1(0), Ψf ∈ TSψ1(T), hΨf , φki = hΨ0, φkie −iλkT for every k ∈ N ∗ such that hµφ1, φki = 0, there exists v ∈ L 2 ((0, T), R) such that the solution of (5.6) satisfies Ψ(T) = Ψf . 5.2. Definitions, notations and statement of the results 121 Remark 5.1. Let us emphasize that the assumption (5.10) is generic with respect to µ ∈ W3,∞((0, 1), R). Indeed, thanks to Baire’s Lemma, it is easy to prove that the property “hµφ1, φki 6= 0, ∀k ∈ N ∗ ”holds generically with respect to µ ∈ W3,∞((0, 1), R). Moreover, for such a function µ, integrations by parts lead to hµφ1, φki = 2 Z 1 0 µ(q) sin(πq) sin(kπq)dq = 4k[(−1)k+1µ ′ (1) − µ ′ (0)] (k 2 − 1)2 + o  1 k 3  . Thus, the asymptotic behavior in 1/k3 of these coefficients is equivalent to the property “µ ′ (1) ± µ ′ (0) 6= 0”, that is also generic in W3,∞((0, 1), R). The key ingredient for the proof of Proposition 5.2 is the following theorem due to Kahane [56, Theorem III.6.1, p. 114]. Theorem 5.3. Let (µk)k∈N∗ ⊂ R such that µ1 = 0 and µk+1 − µk ≥ ρ > 0, ∀k ∈ N ∗ . (5.12) Let T > 0 be such that lim x→+∞ N(x) x < T 2π , where, for x > 0, N(x) is the largest number of µk’s contained in an interval of length x. Then, there exists C > 0 such that, for every c = (ck)k∈N∗ ∈ l 2 (N ∗ , C) with c1 ∈ R, there exists w ∈ L 2 ((0, T), R) such that kwkL2((0,T),R) ≤ Ckckl 2(N∗,C) and Z T 0 w(t)e iµkt dt = ck, ∀k ∈ N ∗ . Remark 5.2. The proof of Theorem 5.3 relies on an Ingham inequality for the family {1, eiµkt , e−iµkt ; k ∈ N ∗ , k ≥ 2}, which corresponds to the Riesz basis property of this family in L 2 ((0, T), C). For the proof of Theorem 5.3, see, for example Krabs [61, Section 1.2.2], Komornik and Loreti [60, Chapter 9], or Avdonin and Ivanov [6, Chapter II Section 4]. For the proof of similar results, we also refer to the prior works by Ingham [48], and to Beurling [14, p. 341-365], Haraux [42], Redheffer [83], Russel [86, Section 3], Schwartz [87]. Improvements of Theorem 5.3 have been obtained by Jaffard, Tucsnak and Zuazua [50], [51], Jaffard and Micu [49], Baiocchi, Komornik and Loreti [7], Komornik and Loreti [59], [60, Theorem 9.4, p. 177]. Proof of Proposition 5.2. We assume (5.10). Let T > 0 and Ψ0 ∈ TSψ1(0). By definition, the weak solution of (5.6) with some control v ∈ L 2 ((0, T), R) is Ψ(t, q) = X∞ k=1 xk(t)φk(q), where xk(t) =  hΨ0, φki + ihµφ1, φki Z t 0 v(τ )e iωkτ dτ e −iλkt , ∀ k ∈ N ∗ , with convergence in L 2 ((0, 1), C) for every t ∈ [0, T], where ωk := λk − λ1, for every k ∈ N ∗ . Since hµφ1, φki 6= 0, for every k ∈ N ∗ , the equality Ψ(T) = Ψf in L 2 ((0, 1), C) is equivalent to the following trigonometric moment problem on the control v, Z T 0 v(t)e iωkt dt = dk, ∀k ∈ N ∗ , (5.13) 122 CHAPITRE 5. SPECTRAL CONTROLLABILITY FOR 2D AND 3D LINEAR SCHRÖDINGER EQUATIONS where dk := hΨf , φkie iλkT − hΨ0, φki ihµφ1, φki , ∀k ∈ N ∗ . (5.14) Thanks to (5.10), the right-hand side (dk)k∈N∗ belongs to l 2 (N ∗ , C) if and only if Ψf − e −iATΨ0 ∈ H3 (0)((0, 1), C), and in that case, (5.13) has a solution v ∈ L 2 ((0, T), R) for every T > 0, thanks to Theorem 5.3. The proof of the statement (2) is similar. Now, let us discuss the generalization of Proposition 5.2 to the 2D and 3D cases. In 2D and 3D, the equality Ψ(T) = Ψf for a solution of (5.6) is equivalent to i D hµφ1, φkiL2(Ω), Z T 0 v(t)e iωkt dtE Rn = hΨf , φkie iλkT − hΨ0, φki, ∀k ∈ N ∗ . (5.15) Thus, the property hµφ1, φki 6= 0, ∀k ∈ N ∗ is still a necessary condition for the controllability of (5.3). Let us assume that this property holds, then (5.15) is satisfied in particular when Z T 0 v(t)e iωkt dt = −i hµφ1, φki |hµφ1, φki|2  hΨf , φkie iλkT − hΨ0, φki  , ∀k ∈ N ∗ . Thus, the controllability of (5.3) can be reduced to the solvability of n trigonometric moment problems on the real valued functions v (1) ,…,v (n) . In 2D, the existence of a regular domain Ω of R 2 such that the eigenvalues of ∆D Ω present a uniform gap (which corresponds to the assumption (5.12)) is an open problem. For general 2D regular domains, we only have Weyl’s Formula, ∃c = c(Ω) > 0, ∃α = α(Ω) ∈ (0, 1) such that ♯{k ∈ N ∗ ; λk ∈ [0, t]} = ct + O(t α ), when t → +∞. This formula is not sufficient to ensure the existence of a uniform gap between the frequencies ωk. Therefore the classical result given in Theorem 5.3 cannot be applied : the controllability of (5.3) is a more difficult problem in 2D than in 1D. In 3D, with Weyl’s formula, ∃c = c(Ω) > 0, ∃α = α(Ω) ∈ (0, 3/2) such that ♯{k ∈ N ∗ ; λk ∈ [0, t]} = ct3/2 + O(t α ), when t → +∞, no uniform gap is possible. Thus, the non controllability of (5.3) is expected. The exact controllability of (5.3) in 2D and 3D being a difficult problem, it is natural to study a weaker controllability property for this system. This is why we investigate its spectral controllability in this article. Notice that the spectral controllability in time T of (5.3) is equivalent to the existence of a solution v ∈ L 2 ((0, T), R n ) of (5.15) for any right hand side with finite support. This remark will be used in the study of the spectral controllability of (5.3) (see Section 5.3.2).